20. Circular Motion
From Mechanics
| Line 8: | Line 8: | ||
| - | 20. Circular Motion | ||
| - | Key Points | + | == '''Key Points''' == |
The diagram shows a particle | The diagram shows a particle | ||
| Line 21: | Line 20: | ||
The position vector for the particle is: | The position vector for the particle is: | ||
| - | |||
<math>\mathbf{r}=\left( r\cos \theta \right)\mathbf{i}+\left( r\sin \theta \right)\mathbf{j}</math> | <math>\mathbf{r}=\left( r\cos \theta \right)\mathbf{i}+\left( r\sin \theta \right)\mathbf{j}</math> | ||
| - | |||
The particle has angular speed | The particle has angular speed | ||
| Line 39: | Line 36: | ||
<math>t</math>: | <math>t</math>: | ||
| - | |||
<math>\mathbf{r}=\left( r\cos \omega t \right)\mathbf{i}+\left( r\sin \omega t \right)\mathbf{j}</math> | <math>\mathbf{r}=\left( r\cos \omega t \right)\mathbf{i}+\left( r\sin \omega t \right)\mathbf{j}</math> | ||
| - | |||
Differentiating the position vector gives the velocity: | Differentiating the position vector gives the velocity: | ||
| - | |||
<math>\mathbf{v}=\left( -r\omega \sin \omega t \right)\mathbf{i}+\left( r\omega \cos \omega t \right)\mathbf{j}</math> | <math>\mathbf{v}=\left( -r\omega \sin \omega t \right)\mathbf{i}+\left( r\omega \cos \omega t \right)\mathbf{j}</math> | ||
| - | |||
The magnitude of the velocity can now be found: | The magnitude of the velocity can now be found: | ||
| - | |||
<math>\begin{align} | <math>\begin{align} | ||
& v=\sqrt{{{\left( -r\omega \sin \omega t \right)}^{2}}+{{\left( r\omega \cos \omega t \right)}^{2}}} \\ | & v=\sqrt{{{\left( -r\omega \sin \omega t \right)}^{2}}+{{\left( r\omega \cos \omega t \right)}^{2}}} \\ | ||
| + | & \\ | ||
& =\sqrt{{{r}^{2}}{{\omega }^{2}}({{\sin }^{2}}\omega t+{{\cos }^{2}}\omega t)} \\ | & =\sqrt{{{r}^{2}}{{\omega }^{2}}({{\sin }^{2}}\omega t+{{\cos }^{2}}\omega t)} \\ | ||
| + | & \\ | ||
& =\sqrt{{{r}^{2}}{{\omega }^{2}}} \\ | & =\sqrt{{{r}^{2}}{{\omega }^{2}}} \\ | ||
& =r\omega | & =r\omega | ||
\end{align}</math> | \end{align}</math> | ||
| - | |||
The particle has speed, | The particle has speed, | ||
| Line 65: | Line 58: | ||
Differentiating the velocity gives the acceleration: | Differentiating the velocity gives the acceleration: | ||
| - | |||
<math>\begin{align} | <math>\begin{align} | ||
| Line 72: | Line 64: | ||
& =-{{\omega }^{2}}\mathbf{r} | & =-{{\omega }^{2}}\mathbf{r} | ||
\end{align}</math> | \end{align}</math> | ||
| - | |||
This shows that the acceleration has magnitude | This shows that the acceleration has magnitude | ||
| Line 84: | Line 75: | ||
and | and | ||
<math>r.</math> | <math>r.</math> | ||
| - | |||
| - | |||
<math>a=r{{\left( \frac{v}{r} \right)}^{2}}=\frac{{{v}^{2}}}{r}</math> | <math>a=r{{\left( \frac{v}{r} \right)}^{2}}=\frac{{{v}^{2}}}{r}</math> | ||
| - | + | '''[[Example 20.1]]''' | |
| - | Example 20.1 | + | |
A coin of mass 30 grams is placed on a turntable which rotates at 90 rpm. The coin is at a distance of 10 cm from the centre of the turntable. Find the magnitude of the coefficient of friction between the coin and the turntable, if the coin is on the point of slipping. | A coin of mass 30 grams is placed on a turntable which rotates at 90 rpm. The coin is at a distance of 10 cm from the centre of the turntable. Find the magnitude of the coefficient of friction between the coin and the turntable, if the coin is on the point of slipping. | ||
| - | Solution | + | '''Solution''' |
The diagram shows the forces acting on the particle, its weight, the normal reaction from the surface and the friction. | The diagram shows the forces acting on the particle, its weight, the normal reaction from the surface and the friction. | ||
| - | |||
[[Image:E20.1.GIF]] | [[Image:E20.1.GIF]] | ||
| - | |||
| - | |||
| - | |||
| - | |||
The acceleration of | The acceleration of | ||
| Line 111: | Line 94: | ||
The weight can be calculated first: | The weight can be calculated first: | ||
| - | |||
<math>W=0.03\times 9.8=0.294\text{ N}</math> | <math>W=0.03\times 9.8=0.294\text{ N}</math> | ||
| - | |||
As the vertical forces balance: | As the vertical forces balance: | ||
| - | |||
<math>R=\text{ }0.\text{294 N}</math> | <math>R=\text{ }0.\text{294 N}</math> | ||
| - | |||
The angular speed must be converted from rpm to | The angular speed must be converted from rpm to | ||
<math>\text{rad }{{\text{s}}^{-1}}</math>. | <math>\text{rad }{{\text{s}}^{-1}}</math>. | ||
| - | |||
<math>\begin{align} | <math>\begin{align} | ||
| Line 153: | Line 131: | ||
| - | Example 20.2 | + | '''[[Example 20.2]]''' |
A car of mass 1200 kg travels round a bend with radius 20 metres at a constant speed of 12 <math>\text{m}{{\text{s}}^{-1}}</math>. Find the magnitude of the friction force acting on the car. Find the minimum possible value of the coefficient of friction between the tyres and the road. | A car of mass 1200 kg travels round a bend with radius 20 metres at a constant speed of 12 <math>\text{m}{{\text{s}}^{-1}}</math>. Find the magnitude of the friction force acting on the car. Find the minimum possible value of the coefficient of friction between the tyres and the road. | ||
| - | Solution | + | '''Solution''' |
The diagram shows the forces acting on the car. | The diagram shows the forces acting on the car. | ||
| Line 174: | Line 152: | ||
\end{align}</math> | \end{align}</math> | ||
| - | |||
Resolving vertically: | Resolving vertically: | ||
| - | |||
<math>R=1200\times 9.8=11760\text{ N}</math> | <math>R=1200\times 9.8=11760\text{ N}</math> | ||
| Line 183: | Line 159: | ||
Then we can use the friction inequality | Then we can use the friction inequality | ||
<math>F\le mR</math>, to give, | <math>F\le mR</math>, to give, | ||
| - | |||
<math>\begin{align} | <math>\begin{align} | ||
| Line 190: | Line 165: | ||
& \mu \ge \text{0}\text{.735 (to 3 sf)} \\ | & \mu \ge \text{0}\text{.735 (to 3 sf)} \\ | ||
\end{align}</math> | \end{align}</math> | ||
| - | |||
So the least value of | So the least value of | ||
<math>\mu </math> | <math>\mu </math> | ||
is 0.735. | is 0.735. | ||
Revision as of 11:25, 21 February 2010
| Theory | Exercises |
Key Points
The diagram shows a particle
which moves with a circular path.
The position vector for the particle is:
 rcos
rcos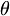
 i+
i+ rsin
rsin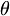
 j
j
The particle has angular speed


If the particle is at
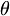 =
= t
t
 rcos
rcos t
t i+
i+ rsin
rsin t
t j
j
Differentiating the position vector gives the velocity:
 −r
−r sin
sin t
t i+
i+ r
r cos
cos t
t j
j
The magnitude of the velocity can now be found:

 −r
−r sin
sin t
t 2+
2+ r
r cos
cos t
t 2=
2= r2
r2 2(sin2
2(sin2 t+cos2
t+cos2 t)=
t)= r2
r2 2=r
2=r
The particle has speed,

Differentiating the velocity gives the acceleration:
 −r
−r 2cos
2cos t
t i+
i+ −r
−r 2sin
2sin t
t j=−
j=−

 rcos
rcos t
t i+
i+ rsin
rsin t
t j
j =−
=− 2r
2r
This shows that the acceleration has magnitude
 2
2
\displaystyle a=r{{\left( \frac{v}{r} \right)}^{2}}=\frac{{{v}^{2}}}{r}
A coin of mass 30 grams is placed on a turntable which rotates at 90 rpm. The coin is at a distance of 10 cm from the centre of the turntable. Find the magnitude of the coefficient of friction between the coin and the turntable, if the coin is on the point of slipping.
Solution
The diagram shows the forces acting on the particle, its weight, the normal reaction from the surface and the friction.
The acceleration of \displaystyle P is horizontal, so the resultant of the vertical forces must be zero.
The weight can be calculated first:
\displaystyle W=0.03\times 9.8=0.294\text{ N}
As the vertical forces balance:
\displaystyle R=\text{ }0.\text{294 N}
The angular speed must be converted from rpm to \displaystyle \text{rad }{{\text{s}}^{-1}}.
\displaystyle \begin{align} & \omega =90\text{ rpm} \\ & =\frac{90\times 2\pi }{60}\text{ rad }{{\text{s}}^{\text{-1}}} \\ & =\text{3}\pi \text{ rad }{{\text{s}}^{\text{-1}}} \end{align}
Using \displaystyle F\text{ }=\text{ }ma in the radial direction with \displaystyle a=r{{\omega }^{2}} gives:
\displaystyle \begin{align} & F=0.03\times 0.1\times {{(3\pi )}^{2}} \\ & =0.267\text{ N} \end{align}
Using \displaystyle F=\mu R gives:
\displaystyle \begin{align} & 0.267=0.294\mu \\ & \mu =\frac{0.267}{0.294}=0.91\text{ (to 2sf)} \\ \end{align}
A car of mass 1200 kg travels round a bend with radius 20 metres at a constant speed of 12 \displaystyle \text{m}{{\text{s}}^{-1}}. Find the magnitude of the friction force acting on the car. Find the minimum possible value of the coefficient of friction between the tyres and the road.
Solution
The diagram shows the forces acting on the car.
Using \displaystyle F=ma radially, with \displaystyle a=\frac{{{v}^{2}}}{r} gives:
\displaystyle \begin{align} & F=1200\times \frac{{{12}^{2}}}{20}\text{ } \\ & =8640\text{ N} \end{align}
Resolving vertically:
\displaystyle R=1200\times 9.8=11760\text{ N}
Then we can use the friction inequality
\displaystyle F\le mR, to give,
\displaystyle \begin{align} & 8640\le \mu \times 11760 \\ & \mu \ge \frac{8640}{11760} \\ & \mu \ge \text{0}\text{.735 (to 3 sf)} \\ \end{align}
So the least value of \displaystyle \mu is 0.735.
