Processing Math: Done

Aus Online Mathematik Brückenkurs 2

Version vom 14:25, 16. Sep. 2008 (bearbeiten) Tekbot (Diskussion | Beiträge) K (Lösning 1.2:2f moved to Solution 1.2:2f: Robot: moved page) ← Zum vorherigen Versionsunterschied		Version vom 13:53, 11. Okt. 2008 (bearbeiten) (rückgängig) Ian (Diskussion | Beiträge) Zum nächsten Versionsunterschied →
Zeile 1:		Zeile 1:
-	{{NAVCONTENT_START}}	+	The entire expression is made up of several levels,
-	<center> [[Image:1_2_2f-1(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+
-	{{NAVCONTENT_START}}	+	<math>\cos \left\{ \left. \sqrt{\left\{ \left. 1-x \right\} \right.} \right\} \right.</math>
-	<center> [[Image:1_2_2f-2(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+
		+	and when we differentiate we go from the outside inwards. In the first stage, we consider the expression as "cos of something",
		+
		+
		+	<math>\cos \left\{ \left. {} \right\} \right.</math>
		+
		+
		+	and differentiate this using the chain rule:
		+
		+
		+	<math>\frac{d}{dx}\cos \left\{ \left. \sqrt{1-x} \right\} \right.=-\sin \left\{ \left. \sqrt{1-x} \right\} \right.\centerdot \left( \left\{ \left. \sqrt{1-x} \right\} \right. \right)^{\prime }</math>
		+
		+
		+	In the next differentiation, we have "the root of something",
		+
		+
		+	<math>\left( \sqrt{\left\{ \left. 1-x \right\} \right.} \right)^{\prime }=\frac{1}{2\sqrt{1-x}}\centerdot \left( 1-x \right)^{\prime }</math>
		+
		+
		+	where we have used the differentiation rule,
		+
		+
		+	<math>\frac{d}{dx}\left( \sqrt{x} \right)=\frac{1}{2\sqrt{x}}</math>
		+
		+
		+	for the outer derivative.
		+
		+	The whole differentiation in one go becomes:
		+
		+
		+	<math>\begin{align}
		+	& \frac{d}{dx}\cos \sqrt{1-x}=-\sin \sqrt{1-x}\centerdot \frac{d}{dx}\sqrt{1-x} \\
		+	& =-\sin \sqrt{1-x}\centerdot \frac{1}{2\sqrt{1-x}}\centerdot \frac{d}{dx}\left( 1-x \right) \\
		+	& =-\sin \sqrt{1-x}\centerdot \frac{1}{2\sqrt{1-x}}\centerdot \left( -1 \right) \\
		+	& =\frac{\sin \sqrt{1-x}}{2\sqrt{1-x}} \\
		+	\end{align}</math>

---

Version vom 13:53, 11. Okt. 2008

The entire expression is made up of several levels,

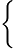
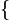cos1−x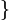 

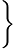
and when we differentiate we go from the outside inwards. In the first stage, we consider the expression as "cos of something",

cos

and differentiate this using the chain rule:

ddxcos1−x=−sin1−x1−x 

In the next differentiation, we have "the root of something",

1−x=121−x1−x 

where we have used the differentiation rule,

ddxx=12x 

for the outer derivative.

The whole differentiation in one go becomes:

ddxcos1−x=−sin1−xddx1−x=−sin1−x121−xddx1−x=−sin1−x121−x−1=21−xsin1−x