Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 1.3:1a

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K
Zeile 1: Zeile 1:
-
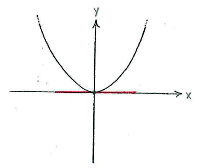
A critical point is a point where the derivative is equal to zero, i.e. the function has a horizontal tangent. For the function in the exercise, this occurs when
+
A critical point is a point where the derivative is equal to zero, i.e. the function has a horizontal tangent. For the function in the exercise, this occurs when <math>x=0</math>.
-
<math>x=0</math>.
+
-
 
+
-
 
+
-
 
+
[[Image:1_3_1_a2.gif|center]]
[[Image:1_3_1_a2.gif|center]]
-
In addition, the point at the origin is a local and global minimum, because no other points give a smaller value for the function that the point at
+
In addition, the point at the origin is a local and global minimum, because no other points give a smaller value for the function than the point at <math>x=0</math>. On the other hand, there are no inflexion points (points where the derivative is both zero and has the same sign on both sides of the point).
-
<math>x=0</math>. On the other hand, there are no inflexion points (points where the derivative is both zero and has the same sign on both sides of the point).
+
-
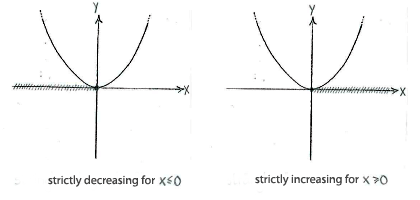
To the left of
+
To the left of <math>x=0</math>, the derivative is negative (the tangent slopes downwards) and the function is strictly decreasing, and to the right of <math>x=0</math> the derivative is positive (the tangent slopes upwards) and the function is strictly increasing.
-
<math>x=0</math>, the derivative is negative (the tangent slopes downwards) and the function is strictly decreasing, and to the right of
+
-
<math>x=0</math>
+
-
the derivative ) the tangent slopes upwards) and the function is strictly increasing.
+
[[Image:1_3_1_a3.gif|center]]
[[Image:1_3_1_a3.gif|center]]

Version vom 11:50, 17. Okt. 2008

A critical point is a point where the derivative is equal to zero, i.e. the function has a horizontal tangent. For the function in the exercise, this occurs when x=0.

In addition, the point at the origin is a local and global minimum, because no other points give a smaller value for the function than the point at x=0. On the other hand, there are no inflexion points (points where the derivative is both zero and has the same sign on both sides of the point).

To the left of x=0, the derivative is negative (the tangent slopes downwards) and the function is strictly decreasing, and to the right of x=0 the derivative is positive (the tangent slopes upwards) and the function is strictly increasing.

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:1a“