Lösung 2.1:2a
Aus Online Mathematik Brückenkurs 2
K (Lösning 2.1:2a moved to Solution 2.1:2a: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | The foremost difficulty with calculating an integral is finding a primitive function of the integrand. Once we have done that, the integral is calculated as the difference between the primitive function's values in the upper and lower limits of integration. |
| - | < | + | |
| - | {{ | + | The integrand in our case consists of two terms in the form |
| - | {{ | + | <math>x^{n}</math>, and so we can use the rule |
| - | < | + | |
| - | {{ | + | |
| + | <math>\int{x^{n}\,dx=\frac{x^{n+1}}{n+1}}+C</math> | ||
| + | |||
| + | |||
| + | on the terms individually to obtain that | ||
| + | |||
| + | |||
| + | <math>F\left( x \right)=\frac{x^{2+1}}{2+1}+3\centerdot \frac{x^{3+1}}{3+1}</math> | ||
| + | |||
| + | |||
| + | is a primitive function of the integrand. | ||
| + | |||
| + | The integrand's value is thus | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & \int\limits_{0}^{2}{\left( x^{2}+3x^{3} \right)}\,dx=\left[ \frac{x^{3}}{3}+3\centerdot \frac{x^{4}}{4} \right]_{0}^{2} \\ | ||
| + | & =\frac{2^{3}}{3}+3\centerdot \frac{2^{4}}{4}-\left( \frac{0^{3}}{3}+3\centerdot \frac{0^{4}}{4} \right) \\ | ||
| + | & =\frac{8}{3}+\frac{3\centerdot 16}{4}=\frac{44}{3} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | NOTE: One way to check that | ||
| + | <math>F\left( x \right)=\frac{1}{3}x^{3}+\frac{3}{4}x^{4}</math> | ||
| + | is a primitive function of the integral is to differentiate | ||
| + | <math>F\left( x \right)</math> | ||
| + | and to see that we obtain | ||
| + | |||
| + | |||
| + | <math>\begin{align} | ||
| + | & {F}'\left( x \right)=\frac{1}{3}\left( x^{3} \right)^{\prime }+\frac{3}{4}\left( x^{4} \right)^{\prime } \\ | ||
| + | & =\frac{1}{3}\centerdot 3x^{2}+\frac{3}{4}\centerdot 4x^{3}=x^{2}+3x^{3} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | as the integrand. | ||
Version vom 12:44, 17. Okt. 2008
The foremost difficulty with calculating an integral is finding a primitive function of the integrand. Once we have done that, the integral is calculated as the difference between the primitive function's values in the upper and lower limits of integration.
The integrand in our case consists of two terms in the form
 xndx=xn+1n+1+C
xndx=xn+1n+1+C
on the terms individually to obtain that
 x
x =x2+12+1+3
=x2+12+1+3 x3+13+1
x3+13+1
is a primitive function of the integrand.
The integrand's value is thus
 20
20 x2+3x3
x2+3x3 dx=
dx= 3x3+3
3x3+3 4x4
4x4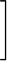 20=323+3
20=323+3 424−
424−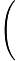 303+3
303+3 404
404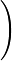 =38+43
=38+43 16=344
16=344
NOTE: One way to check that
 x
x =31x3+43x4
=31x3+43x4  x
x

 x
x =31
=31 x3
x3
 +43
+43 x4
x4
 =31
=31 3x2+43
3x2+43 4x3=x2+3x3
4x3=x2+3x3
as the integrand.
