Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 1.3:2b

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
-
In order to determine the function's extreme points, we investigate three types of points:
+
In order to determine the function's extreme points, we investigate three types of points,
-
1. Critical points, i.e. where
+
# critical points, i.e. where <math>f^{\,\prime}(x)=0</math>,
-
<math>{f}'\left( x \right)=0</math>;
+
# points where the function is not differentiable, and
-
 
+
# endpoints of the interval of definition.
-
2. Points where the function is not differentiable;
+
-
 
+
-
3. Endpoints of the interval of definition.
+
In our case, we have that:
In our case, we have that:
-
1. The derivative of
+
<ol>
-
<math>f\left( x \right)</math>
+
<li>The derivative of <math>f(x)</math> is given by
-
is given by
+
{{Displayed math||<math>f^{\,\prime}(x) = 3-2x</math>}}
-
+
and becomes zero when <math>x=3/2\,</math>.</li>
-
<math>{f}'\left( x \right)=3-2x</math>
+
 
 +
<li>The function is a polynomial, and is therefore differentiable everywhere.</li>
-
and becomes zero when
+
<li>The function is defined for all ''x'', and therefore the interval of definition has no endpoints.</li>
-
<math>x=\frac{3}{2}</math>.
+
</ol>
-
2. The function is a polynomial, and is therefore differentiable everywhere.
+
There is thus just one point <math>x=3/2\,</math>, where the function possibly has an extreme point.
-
3. The function is defined for all
+
If we write down a sign table for the derivative, we see that <math>x=3/2</math> is a local maximum.
-
<math>x</math>, and there are therefore the interval of definition has no
+
-
endpoints.
+
-
There is thus a point
 
-
<math>x=\frac{3}{2}</math>, where the function possibly has an extreme point.
 
-
If we write down a sign table for the derivative, we see that
+
{| border="1" cellpadding="5" cellspacing="0" align="center"
-
<math>x=\frac{3}{2}</math>
+
|-
-
is a local maximum.
+
|width="50px" align="center" style="background:#efefef;"| <math>x</math>
 +
|width="50px" align="center" style="background:#efefef;"|
 +
|width="50px" align="center" style="background:#efefef;"| <math>\tfrac{3}{2}</math>
 +
|width="50px" align="center" style="background:#efefef;"|
 +
|-
 +
|width="50px" align="center"| <math>f^{\,\prime}(x)</math>
 +
|width="50px" align="center"| <math>+</math>
 +
|width="50px" align="center"| <math>0</math>
 +
|width="50px" align="center"| <math>-</math>
 +
|-
 +
|width="50px" align="center"| <math>f(x)</math>
 +
|width="50px" align="center"| <math>\nearrow</math>
 +
|width="50px" align="center"| <math>\tfrac{17}{4}</math>
 +
|width="50px" align="center"| <math>\searrow</math>
 +
|}
-
TABLE
 
-
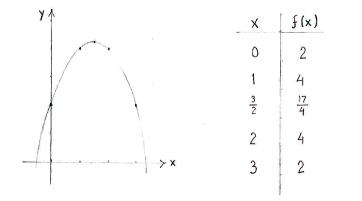
Because the function is given by a second-degree expression, its graph is a parabola with a maximum at
+
Because the function is given by a second-degree expression, its graph is a parabola with a maximum at <math>(3/2, 17/4)</math> and we can draw it with the help of a few couple of points.
-
<math>\left( \frac{3}{2} \right.,\left. \frac{17}{4} \right)</math>
+
-
and we can draw it with the help of a few couple of points.
+
-
PICTURE TABLE
+
[[Image:1_3_2_b.gif||center]]

Version vom 12:46, 17. Okt. 2008

In order to determine the function's extreme points, we investigate three types of points,

  1. critical points, i.e. where f(x)=0,
  2. points where the function is not differentiable, and
  3. endpoints of the interval of definition.

In our case, we have that:

  1. The derivative of f(x) is given by
    f(x)=3−2x
    and becomes zero when x=32.
  2. The function is a polynomial, and is therefore differentiable everywhere.
  3. The function is defined for all x, and therefore the interval of definition has no endpoints.

There is thus just one point x=32, where the function possibly has an extreme point.

If we write down a sign table for the derivative, we see that x=32 is a local maximum.


x 23
f(x) + 0 −
f(x) 417


Because the function is given by a second-degree expression, its graph is a parabola with a maximum at (32174) and we can draw it with the help of a few couple of points.

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:2b“