Processing Math: Done
Lösung 1.2:2e
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 3: | Zeile 3: | ||
To begin with, we have a product of <math>x</math> and <math>(2x+1)^4</math> so the product rule gives that | To begin with, we have a product of <math>x</math> and <math>(2x+1)^4</math> so the product rule gives that | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\frac{d}{dx}\,\bigl[x(2x+1)^4\bigr] | \frac{d}{dx}\,\bigl[x(2x+1)^4\bigr] | ||
&= (x)'\cdot (2x+1)^4 + x\cdot \bigl((2x+1)^4\bigr)'\\[5pt] | &= (x)'\cdot (2x+1)^4 + x\cdot \bigl((2x+1)^4\bigr)'\\[5pt] | ||
| Zeile 11: | Zeile 11: | ||
We can differentiate the expression <math>(2x+1)^4</math> by viewing it as "something raised to the 4", | We can differentiate the expression <math>(2x+1)^4</math> by viewing it as "something raised to the 4", | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,4}\,\textrm{.}</math>}} |
The chain rule then gives | The chain rule then gives | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\frac{d}{dx}\,\bigl[\bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,4}\bigr] | \frac{d}{dx}\,\bigl[\bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,4}\bigr] | ||
&= 4\cdot \bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,3}\cdot \bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,\prime}\,,\\[5pt] | &= 4\cdot \bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,3}\cdot \bbox[#FFEEAA;,1.5pt]{\phantom{(2x+1)}\!\!}^{\,\prime}\,,\\[5pt] | ||
| Zeile 23: | Zeile 23: | ||
We carry out the last differentiation directly, and obtain | We carry out the last differentiation directly, and obtain | ||
| - | {{ | + | {{Abgesetzte Formel||<math>(2x+1)' = 2\,\textrm{.}</math>}} |
If we go through the whole calculation from the beginning, it is | If we go through the whole calculation from the beginning, it is | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\frac{d}{dx}\,\bigl[x(2x+1)^4\bigr] | \frac{d}{dx}\,\bigl[x(2x+1)^4\bigr] | ||
&= (x)'\cdot (2x+1)^4 + x\cdot \bigl((2x+1)^4\bigr)'\\[2pt] | &= (x)'\cdot (2x+1)^4 + x\cdot \bigl((2x+1)^4\bigr)'\\[2pt] | ||
| Zeile 37: | Zeile 37: | ||
Both terms contain a common factor <math>(2x+1)^3</math> which we can take out to get an answer in factorized form, | Both terms contain a common factor <math>(2x+1)^3</math> which we can take out to get an answer in factorized form, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\frac{d}{dx}\,\bigl[x(2x+1)^4\bigr] | \frac{d}{dx}\,\bigl[x(2x+1)^4\bigr] | ||
&= (2x+1)^3\bigl((2x+1)+8x\bigr)\\[5pt] | &= (2x+1)^3\bigl((2x+1)+8x\bigr)\\[5pt] | ||
&= (2x+1)^3(10x+1)\,\textrm{.} | &= (2x+1)^3(10x+1)\,\textrm{.} | ||
\end{align}</math>}} | \end{align}</math>}} | ||
Version vom 12:53, 10. Mär. 2009
One way to differentiate the expression could be to expand
To begin with, we have a product of
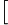 x(2x+1)4 x(2x+1)4 =(x) =(x)  (2x+1)4+x (2x+1)4+x  (2x+1)4 (2x+1)4  =1 =1 (2x+1)4+x (2x+1)4+x  (2x+1)4 (2x+1)4  . . |
We can differentiate the expression
The chain rule then gives
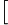 4 4 ddx ddx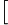 (2x+1)4 (2x+1)4 =4 =4 3 3   =4 =4 (2x+1)3 (2x+1)3 (2x+1) (2x+1) . . |
We carry out the last differentiation directly, and obtain
 =2. =2. |
If we go through the whole calculation from the beginning, it is
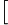 x(2x+1)4 x(2x+1)4 =(x) =(x)  (2x+1)4+x (2x+1)4+x  (2x+1)4 (2x+1)4  =1 =1 (2x+1)4+x (2x+1)4+x 4(2x+1)3 4(2x+1)3 (2x+1) (2x+1) =(2x+1)4+x =(2x+1)4+x 4(2x+1)3 4(2x+1)3 2=(2x+1)4+8x(2x+1)3. 2=(2x+1)4+8x(2x+1)3. |
Both terms contain a common factor
 x(2x+1)4 x(2x+1)4 =(2x+1)3 =(2x+1)3 (2x+1)+8x (2x+1)+8x =(2x+1)3(10x+1). =(2x+1)3(10x+1). |
