Processing Math: Done
Lösung 1.3:2b
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 9: | Zeile 9: | ||
<ol> | <ol> | ||
<li>The derivative of <math>f(x)</math> is given by | <li>The derivative of <math>f(x)</math> is given by | ||
| - | {{ | + | {{Abgesetzte Formel||<math>f^{\,\prime}(x) = 3-2x</math>}} |
and becomes zero when <math>x=3/2\,</math>.</li> | and becomes zero when <math>x=3/2\,</math>.</li> | ||
Version vom 12:55, 10. Mär. 2009
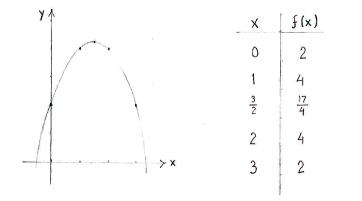
In order to determine the function's extreme points, we investigate three types of points,
- critical points, i.e. where
f , (x)=0
(x)=0 - points where the function is not differentiable, and
- endpoints of the interval of definition.
In our case, we have that:
- The derivative of
f(x) is given by
and becomes zero whenf  (x)=3−2x
(x)=3−2xx=3 . 2
2 - The function is a polynomial, and is therefore differentiable everywhere.
- The function is defined for all x, and therefore the interval of definition has no endpoints.
There is thus just one point  2
2
If we write down a sign table for the derivative, we see that  2
2
| | | ||
 (x) (x) | | | |
| |  | |  |
Because the function is given by a second-degree expression, its graph is a parabola with a maximum at  2
2 17
17 4)
4)