Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 1.3:6

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 1: Zeile 1:
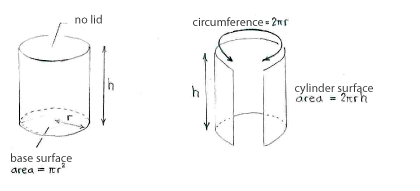
If we call the radius of the metal can ''r'' and its height ''h'', then we can determine the can's volume and area by using the figures below,
If we call the radius of the metal can ''r'' and its height ''h'', then we can determine the can's volume and area by using the figures below,
-
{{Displayed math||<math>\begin{align}
+
{{Abgesetzte Formel||<math>\begin{align}
\text{Volume} &= \text{(area of the base)}\cdot\text{(height)}\\[5pt]
\text{Volume} &= \text{(area of the base)}\cdot\text{(height)}\\[5pt]
&= \pi r^2\cdot h\,,\\[10pt]
&= \pi r^2\cdot h\,,\\[10pt]
Zeile 14: Zeile 14:
From the formula for the volume, we can make ''h'' the subject,
From the formula for the volume, we can make ''h'' the subject,
-
{{Displayed math||<math>h=\frac{V}{\pi r^2}</math>}}
+
{{Abgesetzte Formel||<math>h=\frac{V}{\pi r^2}</math>}}
and express the area solely in terms of the radius, ''r'',
and express the area solely in terms of the radius, ''r'',
-
{{Displayed math||<math>A = \pi r^2 + 2\pi r\cdot\frac{V}{\pi r^2} = \pi r^2 + \frac{2V}{r}\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>A = \pi r^2 + 2\pi r\cdot\frac{V}{\pi r^2} = \pi r^2 + \frac{2V}{r}\,\textrm{.}</math>}}
The minimisation problem is then:
The minimisation problem is then:
Zeile 28: Zeile 28:
The derivative is given by
The derivative is given by
-
{{Displayed math||<math>A'(r) = 2\pi r - \frac{2V}{r^2}\,,</math>}}
+
{{Abgesetzte Formel||<math>A'(r) = 2\pi r - \frac{2V}{r^2}\,,</math>}}
and if we set the derivative equal to zero, so as to obtain the critical points, we get
and if we set the derivative equal to zero, so as to obtain the critical points, we get
-
{{Displayed math||<math>\begin{align}
+
{{Abgesetzte Formel||<math>\begin{align}
& 2\pi r - \frac{2V}{r^2} = 0\quad \Leftrightarrow \quad 2\pi r = \frac{2V}{r^2}\\[5pt]
& 2\pi r - \frac{2V}{r^2} = 0\quad \Leftrightarrow \quad 2\pi r = \frac{2V}{r^2}\\[5pt]
&\quad\Leftrightarrow \quad r^3=\frac{V}{\pi}\quad \Leftrightarrow \quad r=\sqrt[\scriptstyle 3]{\frac{V}{\pi}}\,\textrm{.}
&\quad\Leftrightarrow \quad r^3=\frac{V}{\pi}\quad \Leftrightarrow \quad r=\sqrt[\scriptstyle 3]{\frac{V}{\pi}}\,\textrm{.}
Zeile 39: Zeile 39:
For this value of ''r'', the second derivative,
For this value of ''r'', the second derivative,
-
{{Displayed math||<math>A''(r) = 2\pi + \frac{4V}{r^3}\,,</math>}}
+
{{Abgesetzte Formel||<math>A''(r) = 2\pi + \frac{4V}{r^3}\,,</math>}}
has the value
has the value
-
{{Displayed math||<math>A''\bigl(\sqrt[3]{V/\pi}\bigr) = 2\pi + \frac{4V}{V/\pi } = 6\pi > 0\,,</math>}}
+
{{Abgesetzte Formel||<math>A''\bigl(\sqrt[3]{V/\pi}\bigr) = 2\pi + \frac{4V}{V/\pi } = 6\pi > 0\,,</math>}}
which shows that <math>r=\sqrt[3]{V/\pi}</math> is a local minimum.
which shows that <math>r=\sqrt[3]{V/\pi}</math> is a local minimum.
Zeile 54: Zeile 54:
The metal can has the least area for a given volume <math>V</math> when
The metal can has the least area for a given volume <math>V</math> when
-
{{Displayed math||<math>\begin{align}
+
{{Abgesetzte Formel||<math>\begin{align}
r &= \sqrt[3]{V/\pi}\,,\quad\text{and}\\[5pt]
r &= \sqrt[3]{V/\pi}\,,\quad\text{and}\\[5pt]
h &= \frac{V}{\pi r^{2}} = \frac{V}{\pi}\Bigl(\frac{V}{\pi}\Bigr)^{-2/3} = \Bigl( \frac{V}{\pi}\Bigr)^{1-2/3} = \Bigl(\frac{V}{\pi}\Bigr)^{1/3} = \sqrt[3]{\frac{V}{\pi}}\,\textrm{.}
h &= \frac{V}{\pi r^{2}} = \frac{V}{\pi}\Bigl(\frac{V}{\pi}\Bigr)^{-2/3} = \Bigl( \frac{V}{\pi}\Bigr)^{1-2/3} = \Bigl(\frac{V}{\pi}\Bigr)^{1/3} = \sqrt[3]{\frac{V}{\pi}}\,\textrm{.}
\end{align}</math>}}
\end{align}</math>}}

Version vom 12:57, 10. Mär. 2009

If we call the radius of the metal can r and its height h, then we can determine the can's volume and area by using the figures below,

VolumeArea=(area of the base)(height)=r2h=(area of the base)+(area of the cylindrical surface)=r2+2rh.

The problem can then be formulated as: minimise the can's area, A=r2+2h, whilst at the same time keeping the volume, V=r2h, constant.

From the formula for the volume, we can make h the subject,

h=Vr2

and express the area solely in terms of the radius, r,

A=r2+2rVr2=r2+r2V.

The minimisation problem is then:

Minimise the area A(r)=r2+r2V, when r0.

The area function A(r) is differentiable for all r0 and the region of definition r0 has no endpoints (r=0 does not satisfy r0), so the function can only assume extreme values at critical points.

The derivative is given by

A(r)=2r−r22V

and if we set the derivative equal to zero, so as to obtain the critical points, we get

2r−r22V=02r=r22Vr3=Vr=3V.

For this value of r, the second derivative,

A(r)=2+r34V

has the value

A3V=2+4VV=60 

which shows that r=3V  is a local minimum.

Because the region of definition, r0, is open (the endpoint r=0 is not included) and unlimited, we cannot directly say that the area is least when r=3V ; it could be the case that area becomes smaller when r0 or r. In this case, however, the area increases without bound as r0 or r, so r=3V  really is a global minimum.

The metal can has the least area for a given volume V when

rh=3Vand=Vr2=VV−23=V1−23=V13=3V. 
Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_1.3:6“