Processing Math: Done
Lösung 2.1:2c
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
If we recall that <math>\sqrt{x} = x^{1/2}</math>, the integral can be written as | If we recall that <math>\sqrt{x} = x^{1/2}</math>, the integral can be written as | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\int\limits_{4}^{9} \bigl(\sqrt{x}-\frac{1}{\sqrt{x}}\Bigr)\,dx | \int\limits_{4}^{9} \bigl(\sqrt{x}-\frac{1}{\sqrt{x}}\Bigr)\,dx | ||
&= \int\limits_{4}^{9}\Bigl( x^{1/2}-\frac{1}{x^{1/2}}\Bigr)\,dx\\[5pt] | &= \int\limits_{4}^{9}\Bigl( x^{1/2}-\frac{1}{x^{1/2}}\Bigr)\,dx\\[5pt] | ||
| Zeile 11: | Zeile 11: | ||
We obtain | We obtain | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\int\limits_{4}^{9} \bigl( x^{1/2}-x^{-1/2}\bigr)\,dx | \int\limits_{4}^{9} \bigl( x^{1/2}-x^{-1/2}\bigr)\,dx | ||
&= \Bigl[\ \frac{x^{1/2+1}}{1/2+1} - \frac{x^{-1/2+1}}{-1/2+1}\ \Bigr]_{4}^{9}\\[5pt] | &= \Bigl[\ \frac{x^{1/2+1}}{1/2+1} - \frac{x^{-1/2+1}}{-1/2+1}\ \Bigr]_{4}^{9}\\[5pt] | ||
Version vom 12:58, 10. Mär. 2009
If we recall that  x=x1
x=x1 2
2
 94 94  x−1 x−1 x x dx= dx= 94 94 x1 x1 2−1x1 2−1x1 2 2 dx= dx= 94 94 x1 x1 2−x−1 2−x−1 2 2 dx. dx. |
This is a standard integral in which the integrand consists of two terms looking like  2
2 2
2
We obtain
 94 94 x1 x1 2−x−1 2−x−1 2 2 dx= dx= x1 x1 2+11 2+11 2+1−x−1 2+1−x−1 2+1−1 2+1−1 2+1 2+1 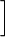 94= 94= 3 3 2x1+1 2x1+1 2−1 2−1 2x1 2x1 2 2 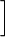 94= 94= 32x 32x x−2 x−2 x x 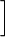 94=32 94=32 9 9  9−2 9−2 9− 9− 32 32 4 4  4−2 4−2 4 4 =32 =32 9 9 3−2 3−2 3− 3− 32 32 4 4 2−2 2−2 2 2 =18−6−316+4=16−316=316 =18−6−316+4=16−316=316 3−16=332. 3−16=332. |
