Processing Math: Done
Lösung 2.1:2d
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
If we rewrite <math>\sqrt{x}</math> as <math>x^{1/2}</math>, the integrand can then be simplified using the power laws, | If we rewrite <math>\sqrt{x}</math> as <math>x^{1/2}</math>, the integrand can then be simplified using the power laws, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\int\limits_1^4 \frac{\sqrt{x}}{x^2}\,dx = \int\limits_1^4 \frac{x^{1/2}}{x^2}\,dx = \int\limits_1^4 x^{1/2-2}\,dx = \int\limits_1^4 x^{-3/2}\,dx\,\textrm{.}</math>}} |
We can now use the fact that a primitive function for <math>x^{n}</math> is <math>x^{n+1}/(n+1)</math> and calculate the integral's value, | We can now use the fact that a primitive function for <math>x^{n}</math> is <math>x^{n+1}/(n+1)</math> and calculate the integral's value, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\int\limits_1^4 x^{-3/2}\,dx | \int\limits_1^4 x^{-3/2}\,dx | ||
&= \Bigl[\ \frac{x^{-3/2+1}}{-3/2+1}\ \Bigr]_1^4\\[5pt] | &= \Bigl[\ \frac{x^{-3/2+1}}{-3/2+1}\ \Bigr]_1^4\\[5pt] | ||
Version vom 12:58, 10. Mär. 2009
If we rewrite  x
x  2
2
 41x2 41x2 xdx= xdx= 41x2x1 41x2x1 2dx= 2dx= 41x1 41x1 2−2dx= 2−2dx= 41x−3 41x−3 2dx. 2dx. |
We can now use the fact that a primitive function for  (n+1)
(n+1)
 41x−3 41x−3 2dx= 2dx= x−3 x−3 2+1−3 2+1−3 2+1 2+1 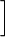 41= 41= −1 −1 2x−1 2x−1 2 2 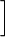 41= 41= −21x1 −21x1 2 2 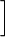 41= 41= −2 −2 x x 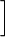 41=−2 41=−2 4− 4− −2 −2 1 1 =−22+2=1. =−22+2=1. |
