Processing Math: Done
Lösung 2.2:2b
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
If we set <math>u=2x+3</math>, the integral simplifies to <math>e^u</math>. However, this is only part of the truth. We must in addition take account of the relation between the integration element <math>dx</math> and <math>du</math>, which can give undesired effects. In this case, however, we have | If we set <math>u=2x+3</math>, the integral simplifies to <math>e^u</math>. However, this is only part of the truth. We must in addition take account of the relation between the integration element <math>dx</math> and <math>du</math>, which can give undesired effects. In this case, however, we have | ||
| - | {{ | + | {{Abgesetzte Formel||<math>du = (2x+3)'\,dx = 2\,dx</math>}} |
which only affects by a constant factor, so the substitution <math>u = 2x+3</math> seems to work, in spite of everything, | which only affects by a constant factor, so the substitution <math>u = 2x+3</math> seems to work, in spite of everything, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\int\limits_0^{1/2} e^{2x+3}\,dx &= \left\{\begin{align} | \int\limits_0^{1/2} e^{2x+3}\,dx &= \left\{\begin{align} | ||
u &= 2x+3\\[5pt] | u &= 2x+3\\[5pt] | ||
Version vom 13:01, 10. Mär. 2009
If we set
 dx=2dx dx=2dx |
which only affects by a constant factor, so the substitution
 1 1 20e2x+3dx= 20e2x+3dx= udu=2x+3=2dx udu=2x+3=2dx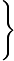 =21 =21 43eudu=21 43eudu=21 eu eu 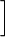 43=21 43=21 e4−e3 e4−e3 . . |
Note: Another possible substitution is
