Processing Math: Done
Lösung 2.2:2c
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
If we focus on the integrand, then the substitution <math>u=3x+1</math> seems suitable, since we then get <math>\sqrt{u}</math> which we can integrate. There is also no risk involved in using a linear substitution such as <math>u=3x+1</math>, because the relation between <math>dx</math> and <math>du</math> will be a constant factor, | If we focus on the integrand, then the substitution <math>u=3x+1</math> seems suitable, since we then get <math>\sqrt{u}</math> which we can integrate. There is also no risk involved in using a linear substitution such as <math>u=3x+1</math>, because the relation between <math>dx</math> and <math>du</math> will be a constant factor, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>du = (3x+1)'\,dx = 3\,dx\,,</math>}} |
which does not cause any problems. | which does not cause any problems. | ||
| Zeile 7: | Zeile 7: | ||
We obtain | We obtain | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\int\limits_0^5 \sqrt{3x+1}\,dx | \int\limits_0^5 \sqrt{3x+1}\,dx | ||
&= \left\{\begin{align} | &= \left\{\begin{align} | ||
Version vom 13:01, 10. Mär. 2009
If we focus on the integrand, then the substitution  u
u
 dx=3dx dx=3dx |
which does not cause any problems.
We obtain
 50 50 3x+1dx= 3x+1dx= udu=3x+1=3dx udu=3x+1=3dx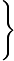 =31 =31 161 161 udu=31 udu=31 161u1 161u1 2du=31 2du=31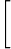 21+1u1 21+1u1 2+1 2+1  116=31 116=31 32u 32u u u 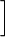 116=92 116=92 16 16 16−1 16−1 1 1 =92 =92 16 16 4−1 4−1 =92 =92 63=14. 63=14. |
