Lösung 2.1:4c
Aus Online Mathematik Brückenkurs 2
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (Solution 2.1:4c moved to Lösung 2.1:4c: Robot: moved page) |
Version vom 10:18, 11. Mär. 2009
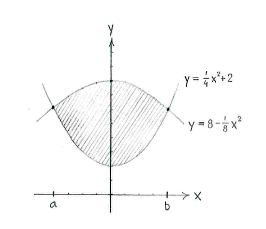
First, we need a picture of what the region looks like.
Both curves,  4+2
4+2 8
8
The region is bounded above by the parabola  8
8 4+2
4+2
 ba ba  8−81x2 8−81x2 − − 41x2+2 41x2+2  dx. dx. |
The integrand is the y-value for the upper parabola minus the corresponding y-value for the lower parabola.
At the points where the curves intersect each other, the x- and y-coordinates are equal, which gives the equation system,
   yy=8−81x2 yy=8−81x2 =41x2+2. =41x2+2. |
If we eliminate y from this system, we get the following equation for x,
If we move the
 |
i.e.
 41+81 41+81 x283x2x2=6 x283x2x2=6 =6 =6 =16. =16. |
The x-coordinates of the points of intersection are therefore equal to
The area of the area between the curves is given by
 4−4 4−4  8−81x2 8−81x2 − − 41x2+2 41x2+2  dx= dx= 4−4 4−4 8−81x2−41x2−2 8−81x2−41x2−2 dx= dx= 4−4 4−4 6− 6− 81+41 81+41 x2 x2 dx= dx= 4−4 4−4 6−83x2 6−83x2 dx= dx= 6x−833x3 6x−833x3 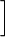 4−4= 4−4= 6x−8x3 6x−8x3 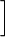 4−4=6 4−4=6 4−843− 4−843− 6 6 (−4)−8(−4)3 (−4)−8(−4)3 =24−8+24−8=32. =24−8+24−8=32. |