Processing Math: Done
Lösung 2.3:2a
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (Solution 2.3:2a moved to Lösung 2.3:2a: Robot: moved page) |
Version vom 10:27, 11. Mär. 2009
Had the integral instead been
 e e x x 12 12 xdx xdx |
it is quite obvious that we would substitute  x
x  2
2 x
x  x
x
 e e xdx= xdx= e e x x 2 2 x x 12 12 xdx= xdx=     udu= udu= x= x=  x x  dx=12 dx=12 xdx xdx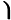 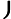 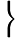   = = eu eu 2udu. 2udu. |
Now, we obtain instead another, not entirely simple, integral, but we can calculate the new integral by partial integration (
 eu eu 2udu=eu 2udu=eu 2u− 2u− eu eu 2du=2ueu−2 2du=2ueu−2 eudu=2ueu−2eu+C=2(u−1)eu+C. eudu=2ueu−2eu+C=2(u−1)eu+C. |
If we substitute back  x
x
 e e xdx=2( xdx=2( x−1)e x−1)e x+C. x+C. |
As can be seen, it is possible to mix different integration techniques and often we need to experiment with different approaches before we find the right one.
