Lösung 2.3:2b
Aus Online Mathematik Brückenkurs 2
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (Solution 2.3:2b moved to Lösung 2.3:2b: Robot: moved page) |
Version vom 10:27, 11. Mär. 2009
We have a product of two factors in the integrand, so an integration by parts does not seem unreasonable. There is nevertheless a problem as regards which factor should be differentiated and which should be integrated. If we choose to differentiate
 x3 x3 ex2dx=4x4 ex2dx=4x4 ex2− ex2− 4x4 4x4 ex22xdx=41x4ex2−21 ex22xdx=41x4ex2−21 x5ex2dx x5ex2dx |
which just seems to make the integral harder. The solution is instead to substitute
 10x3ex2dx= 10x3ex2dx= 10x2ex2xdx 10x2ex2xdx |
we see that the expression "
 10x3ex2dx= 10x3ex2dx= 10x2ex2xdx= 10x2ex2xdx= udu=x2= udu=x2= x2 x2  dx=2xdx dx=2xdx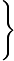 = = 10ueu21du=21 10ueu21du=21 10ueudu. 10ueudu. |
We can then calculate this integral by integration by parts, where we differentiate away the factor
 10ueudu=21 10ueudu=21 ueu ueu 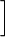 10−21 10−21 101 101 eudu=21 eudu=21 1 1 e1−0 e1−0 −21 −21 eu eu 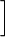 10=21e−21 10=21e−21 e1−e0 e1−e0 =21e−21e+21=21. =21e−21e+21=21. |
