Processing Math: Done
Lösung 2.1:2a
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K (Robot: Automated text replacement (-[[Bild: +[[Image:)) |
|||
| (Der Versionsvergleich bezieht 7 dazwischen liegende Versionen mit ein.) | |||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | Die Schwierigkeit in der Integralrechnung liegt darin, eine Stammfunktion zu finden. Danach müssen wir nur die Stammfunktion in den beiden Integrationzgrenzen evaluieren. |
| - | < | + | |
| - | {{ | + | Da unser Integrand in der Form <math>x^n</math> ist, können wir die Regel |
| - | {{ | + | |
| - | < | + | {{Abgesetzte Formel||<math>\int x^n\,dx = \frac{x^{n+1}}{n+1}+C</math>}} |
| - | {{ | + | |
| + | für jeden Term benutzen. | ||
| + | |||
| + | {{Abgesetzte Formel||<math>F(x) = \frac{x^{2+1}}{2+1} + 3\cdot \frac{x^{3+1}}{3+1}</math>}} | ||
| + | |||
| + | Der Wert des Integrals ist daher | ||
| + | |||
| + | {{Abgesetzte Formel||<math>\begin{align} | ||
| + | \int\limits_{0}^{2} \bigl( x^2+3x^3\bigr)\,dx | ||
| + | &= \Bigl[\ \frac{x^3}{3} + 3\cdot\frac{x^4}{4}\Bigr]_0^2\\ | ||
| + | &= \frac{2^3}{3} + 3\cdot\frac{2^4}{4} - \Bigl(\frac{0^3}{3} + 3\cdot\frac{0^4}{4} \Bigr)\\[5pt] | ||
| + | &= \frac{8}{3} + \frac{3\cdot 16}{4}\\[5pt] | ||
| + | &= \frac{44}{3}\,\textrm{.} | ||
| + | \end{align}</math>}} | ||
| + | |||
| + | |||
| + | Hinweis: Wir können testen ob <math>F(x) = \tfrac{1}{3}x^3 + \tfrac{3}{4}x^4</math> eine Stammfunktion von dem Integrand ist, indem wir <math>F(x)</math> ableiten | ||
| + | |||
| + | {{Abgesetzte Formel||<math>\begin{align} | ||
| + | F'(x) &= \tfrac{1}{3}\bigl(x^3\bigr)' + \tfrac{3}{4}\bigl(x^4\bigr)'\\[5pt] | ||
| + | &= \tfrac{1}{3}\cdot 3x^2 + \tfrac{3}{4}\cdot 4x^3\\[5pt] | ||
| + | &= x^2+3x^3\,\textrm{.} | ||
| + | \end{align}</math>}} | ||
Aktuelle Version
Die Schwierigkeit in der Integralrechnung liegt darin, eine Stammfunktion zu finden. Danach müssen wir nur die Stammfunktion in den beiden Integrationzgrenzen evaluieren.
Da unser Integrand in der Form
 xndx=xn+1n+1+C xndx=xn+1n+1+C |
für jeden Term benutzen.
 x3+13+1 x3+13+1 |
Der Wert des Integrals ist daher
 20 20 x2+3x3 x2+3x3 dx= dx= 3x3+3 3x3+3 4x4 4x4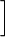 20=323+3 20=323+3 424− 424− 303+3 303+3 404 404 =38+43 =38+43 16=344. 16=344. |
Hinweis: Wir können testen ob
 (x)=31 (x)=31 x3 x3  +43 +43 x4 x4  =31 =31 3x2+43 3x2+43 4x3=x2+3x3. 4x3=x2+3x3. |
