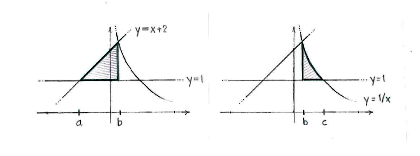Processing Math: 78%
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 2.1:4d

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (11:14, 27. Aug. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht eine dazwischen liegende Version mit ein.)
Zeile 14: Zeile 14:
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
-
\text{Linke Fläche} &= \int\limits_a^b (x+2-1)\,dx\,,\\[5pt]
+
\text{Linke Fläche} &= \int\limits_a^b (x+2-1)\,dx\,\\[5pt]
-
\text{Rechte Fläche} &= \int\limits_b^c \Bigl(\frac{1}{x}-1\Bigr)\,dx\,,
+
\text{Rechte Fläche} &= \int\limits_b^c \Bigl(\frac{1}{x}-1\Bigr)\,dx\,
\end{align}</math>}}
\end{align}</math>}}
-
und die gesamte Fläche ist die Summe der beiden Flächen.
+
Die gesamte Fläche ist die Summe der beiden Flächen.
Wir suchen also die Schnittstellen:
Wir suchen also die Schnittstellen:
Zeile 38: Zeile 38:
\end{align}\right.</math>}}
\end{align}\right.</math>}}
-
:Eliminieren wir <math>y</math> erhalten wir eine Gleichung für <math>x</math>,
+
:Eliminieren wir <math>y</math> erhalten wir für <math>x</math> diese Gleichung
{{Abgesetzte Formel||<math>x+2=\frac{1}{x}\,,</math>}}
{{Abgesetzte Formel||<math>x+2=\frac{1}{x}\,,</math>}}
Zeile 49: Zeile 49:
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
-
(x+1)^2 - 1^2 &= 1\,,\\[5pt]
+
(x+1)^2 - 1^2 &= 1\,\\[5pt]
-
(x+1)^2 &= 2\,,
+
(x+1)^2 &= 2\,.
\end{align}</math>}}
\end{align}</math>}}
-
:Die Wurzeln sind daher <math>x=-1\pm \sqrt{2}</math>, und dies ergibt
+
:Die Wurzeln sind daher <math>x=-1\pm \sqrt{2}</math> und dies ergibt
<math>b=-1+\sqrt{2}</math>. (Die Lösung <math>b=-1-\sqrt{2}</math>
<math>b=-1+\sqrt{2}</math>. (Die Lösung <math>b=-1-\sqrt{2}</math>
liegt links von <math>x=a\,</math>.)
liegt links von <math>x=a\,</math>.)
-
*<math>x=c</math>: Dies ist die Schnittstelle von <math>y=1</math> und <math>y=1/x\,</math>, also ist <math>x=1\,</math>, und daher <math>c=1\,</math>.
+
*<math>x=c</math>: Dies ist die Schnittstelle von <math>y=1</math> und <math>y=1/x\,</math>, also ist <math>x=1\,</math>, daher <math>c=1\,</math>.
Zeile 72: Zeile 72:
&= \frac{2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
&= \frac{2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
&= 1 - \sqrt{2} + \frac{1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
&= 1 - \sqrt{2} + \frac{1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt]
-
&= 1\.\\[10pt]
+
&= 1\,\textrm{.}\\[10pt]
\text{Rechte Fläche}
\text{Rechte Fläche}
&= \int\limits_{\sqrt{2}-1}^1 \Bigl(\frac{1}{x}-1\Bigr)\,dx\\[5pt]
&= \int\limits_{\sqrt{2}-1}^1 \Bigl(\frac{1}{x}-1\Bigr)\,dx\\[5pt]
Zeile 81: Zeile 81:
\end{align}</math>}}
\end{align}</math>}}
-
und die gesamte Fläche ist
+
Die gesamte Fläche ist
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}

Aktuelle Version

Wir zeichnen die Kurven.

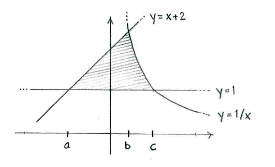
Zeichnen wir alle Kurven im selben Bild, sehen wir, dass die Fläche unten von der Geraden y=1 begrenzt ist und oben von den Kurven y=x+2 und y=1x.

Wir benennen die drei Schnittstellen der Kurven x=a, x=b und x=c, wie in der Figur gezeigt. Wir sehen so, dass die Fläche in zwei Teilflächen eingeteilt werden kann. Eine zwischen x=a und x=b, wo die obere Grenze y=x+2 ist und eine zwischen x=b und x=c wo y=1x die obere Grenze ist.

Die Flächen dieser Gebiete sind

Linke FlächeRechte Fläche=ba(x+2−1)dx=cbx1−1dx

Die gesamte Fläche ist die Summe der beiden Flächen.

Wir suchen also die Schnittstellen:

  • x=a: Die Schnittstelle von y=1 und y=x+2 erfüllt beide Gleichungen:
yy=1=x+2. 
Dies ergibt x+2=1, also x=−1. Daher ist a=−1.


  • x=b: Die Schnittstelle von y=x+2 und y=1x erfüllt beide Gleichungen:
yy=x+2=1x. 
Eliminieren wir y erhalten wir für x diese Gleichung
x+2=x1
die wir mit x multiplizieren,
x2+2x=1.
Quadratische Ergänzung ergibt:
(x+1)2−12(x+1)2=1=2
Die Wurzeln sind daher x=−12  und dies ergibt

b=−1+2 . (Die Lösung \displaystyle b=-1-\sqrt{2} liegt links von \displaystyle x=a\,.)


  • \displaystyle x=c: Dies ist die Schnittstelle von \displaystyle y=1 und \displaystyle y=1/x\,, also ist \displaystyle x=1\,, daher \displaystyle c=1\,.


Die Teilflächen sind also

\displaystyle \begin{align}

\text{Linke Fläche} &= \int\limits_{-1}^{\sqrt{2}-1} (x+2-1)\,dx\\[5pt] &= \int\limits_{-1}^{\sqrt{2}-1} (x+1)\,dx\\[5pt] &= \Bigl[\ \frac{x^2}{2} + x\ \Bigr]_{-1}^{\sqrt{2}-1}\\[5pt] &= \frac{\bigl(\sqrt{2}-1\bigr)^2}{2} + \sqrt{2} - 1 - \Bigl(\frac{(-1)^2}{2} + (-1) \Bigr)\\[5pt] &= \frac{\bigl(\sqrt{2}\bigr)^2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt] &= \frac{2-2\sqrt{2}+1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt] &= 1 - \sqrt{2} + \frac{1}{2} + \sqrt{2} - 1 - \frac{1}{2} + 1\\[5pt] &= 1\,\textrm{.}\\[10pt] \text{Rechte Fläche} &= \int\limits_{\sqrt{2}-1}^1 \Bigl(\frac{1}{x}-1\Bigr)\,dx\\[5pt] &= \Bigl[\ \ln |x| - x\ \Bigr]_{\sqrt{2}-1}^1\\[5pt] &= \ln 1 - 1 - \Bigl( \ln \bigl(\sqrt{2}-1\bigr)-\bigl(\sqrt{2}-1\bigr)\Bigr)\\[5pt] &= 0 - 1 - \ln \bigl(\sqrt{2}-1\bigr) + \sqrt{2} - 1\\[5pt] &= \sqrt{2} - 2 - \ln\bigl(\sqrt{2}-1\bigr)\,\textrm{.} \end{align}

Die gesamte Fläche ist

\displaystyle \begin{align}

\text{Fläche} &= \text{(Linke Fläche)} + \text{(Rechte Fläche)}\\[5pt] &= 1 + \sqrt{2} - 2 - \ln\bigl(\sqrt{2}-1\bigr)\\[5pt] &= \sqrt{2} - 1 - \ln\bigl(\sqrt{2}-1\bigr)\,\textrm{.} \end{align}

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_2.1:4d“