Lösung 1.3:4
Aus Online Mathematik Brückenkurs 2
K (Robot: Automated text replacement (-ä +ä)) |
|||
| Zeile 29: | Zeile 29: | ||
{{Abgesetzte Formel||<math>A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,</math>}} | {{Abgesetzte Formel||<math>A''\bigl( 1/\!\sqrt{3}\bigr) = -6\cdot\frac{1}{\sqrt{3}} < 0\,,</math>}} | ||
| - | also hat die | + | also hat die Flächenfunktion an der Stelle <math>x=1/\!\sqrt{3}</math> ein lokales Maximum. |
Also ist der optimale Punkt <math>P</math> | Also ist der optimale Punkt <math>P</math> | ||
{{Abgesetzte Formel||<math>P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}</math>}} | {{Abgesetzte Formel||<math>P = \Bigl(\frac{1}{\sqrt{3}}, 1-\Bigl(\frac{1}{\sqrt{3}} \Bigr)^2\, \Bigr) = \Bigl(\frac{1}{\sqrt{3}}, \frac{2}{3} \Bigr)\,\textrm{.}</math>}} | ||
Aktuelle Version
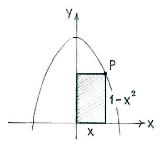
Wir nennen die x-Koordinate des Punktes
Die Fläche des Rechtecks ist
 (Höhe)=x(1−x2) |
Wir wollen diese Fläche maximieren.
Wir sehen, dass 
00
1x1
Lokale Extremstelle der Fläche sind entweder:
- stationäre Stellen mit
f ,
(x)=0 - singuläre Stellen, in denen die Funktion nicht differenzierbar ist, oder
- Randstellen.
Die Funktion
Die Ableitung der Funktion ist
| (x)=1(1−x2)+x(−2x)=1−3x2 |
und wir erhalten die Gleichung 
1
3
Nur die Lösung 3 x1
Die zweite Ableitung (x)=−6x
 13 =−613 0 |
also hat die Flächenfunktion an der Stelle 3
Also ist der optimale Punkt
 131−13 2=1332. |
