Lösung 2.1:1b

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Aktuelle Version (07:33, 21. Aug. 2009) (bearbeiten) (rückgängig)
 
(Der Versionsvergleich bezieht 4 dazwischen liegende Versionen mit ein.)
Zeile 1: Zeile 1:
-
The graph of the function
+
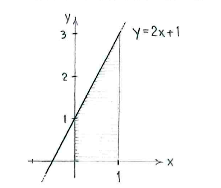
Die Funktion <math>y=2x+1</math> ist eine Gerade, die die ''y''-Achse in <math>y=1</math> schneidet und die Steigung 2 hat.
-
<math>y=2x+1</math>
+
-
is a straight line which cuts the
+
-
<math>y</math>
+
-
-axis at
+
-
<math>y=\text{1}</math>
+
-
and has gradient
+
-
<math>2</math>.
+
-
 
+
-
The integral's value is the area under the straight line and between
+
-
<math>x=0\text{ }</math>
+
-
and
+
-
<math>x=\text{1}</math>.
+
-
 
+
 +
Das Integral entspricht der Fläche unter der Geraden zwischen <math>x=0</math>
 +
und <math>x=1</math>.
[[Image:2_1_1_b1.gif|center]]
[[Image:2_1_1_b1.gif|center]]
-
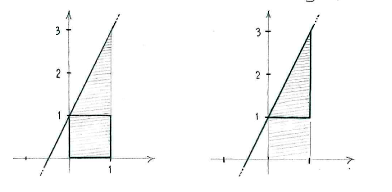
We can divide up the region under the graph into a square and rectangle,
+
Wir teilen die Fläche in zwei Teilflächen auf, ein Rechteck und ein Dreieck
-
 
+
[[Image:2_1_1_b2.gif|center]]
[[Image:2_1_1_b2.gif|center]]
-
and then add up the area to obtain the total area.
+
und addieren deren Flächen, um die gesamte Fläche zu bekommen.
-
 
+
-
The value of the integral is
+
 +
Das Integral ist daher
-
<math>\int\limits_{0}^{1}{\left( 2x+1 \right)\,}dx=</math>
+
{{Abgesetzte Formel||<math>\begin{align}
-
(area of the square) + (area of the triangle)
+
\int\limits_{0}^{1} (2x+1)\,dx
-
<math>1\centerdot 1+\frac{1}{2}\centerdot 1\centerdot 2=2</math>
+
&= \text{(Fläche des Rechtecks)} + \text{(Fläche des Dreiecks)}\\
 +
&= 1\cdot 1 + \frac{1}{2}\cdot 1\cdot 2 = 2\,\textrm{.}
 +
\end{align}</math>}}

Aktuelle Version

Die Funktion \displaystyle y=2x+1 ist eine Gerade, die die y-Achse in \displaystyle y=1 schneidet und die Steigung 2 hat.

Das Integral entspricht der Fläche unter der Geraden zwischen \displaystyle x=0 und \displaystyle x=1.

Wir teilen die Fläche in zwei Teilflächen auf, ein Rechteck und ein Dreieck

und addieren deren Flächen, um die gesamte Fläche zu bekommen.

Das Integral ist daher

\displaystyle \begin{align}

\int\limits_{0}^{1} (2x+1)\,dx &= \text{(Fläche des Rechtecks)} + \text{(Fläche des Dreiecks)}\\ &= 1\cdot 1 + \frac{1}{2}\cdot 1\cdot 2 = 2\,\textrm{.} \end{align}

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_2.1:1b“