Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 3.2:3

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 3.2:3 moved to Solution 3.2:3: Robot: moved page)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
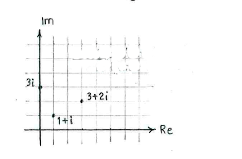
If we mark the three complex numbers in the plane, we see that the fourth corner will have
-
<center> [[Image:3_2_3-1(2).gif]] </center>
+
<math>\text{3}+\text{2}i</math>
-
{{NAVCONTENT_STOP}}
+
and
-
{{NAVCONTENT_START}}
+
<math>\text{3}i</math>
-
<center> [[Image:3_2_3-2(2).gif]] </center>
+
as neighbouring corners.
-
{{NAVCONTENT_STOP}}
+
 
[[Image:3_2_3_1.gif|center]]
[[Image:3_2_3_1.gif|center]]
 +
 +
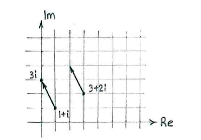
In order to find the fourth corner, we use the fact that in a square opposite sides are parallel and all sides have the same length. This means that the vector from
 +
<math>\text{1}+i</math>
 +
to
 +
<math>\text{3}i</math>
 +
is equal to the vector from
 +
<math>\text{3}+\text{2}i</math>
 +
to the fourth corner.
 +
 +
[[Image:3_2_3_2.gif|center]]
[[Image:3_2_3_2.gif|center]]
 +
 +
If we interpret the complex numbers as vectors, this means that the vector from
 +
<math>\text{1}+i</math>
 +
to
 +
<math>\text{3}i</math>
 +
is
 +
 +
 +
<math>3i-\left( 1+i \right)=-1+2i</math>
 +
 +
 +
And we obtain the fourth corner if we add this vector to the corner
 +
<math>\text{3}+\text{2}i</math>,
 +
 +
 +
<math>\text{3}+\text{2}i+\left( -1+2i \right)=2+4i</math>

Version vom 15:12, 22. Okt. 2008

If we mark the three complex numbers in the plane, we see that the fourth corner will have 3+2i and 3i as neighbouring corners.


In order to find the fourth corner, we use the fact that in a square opposite sides are parallel and all sides have the same length. This means that the vector from 1+i to 3i is equal to the vector from 3+2i to the fourth corner.


If we interpret the complex numbers as vectors, this means that the vector from 1+i to 3i is


3i−1+i=−1+2i 


And we obtain the fourth corner if we add this vector to the corner 3+2i,


3+2i+−1+2i=2+4i 

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.2:3“