Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
jsMath

Lösung 3.3:2c

Aus Online Mathematik Brückenkurs 2

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Solution 3.3:2c moved to Lösung 3.3:2c: Robot: moved page)
Zeile 1: Zeile 1:
-
We write <math>z</math> and the right-hand side <math>-1-i</math> in polar form
+
Wir bringen <math>z</math> und <math>-1-i</math> auf Polarform
{{Abgesetzte Formel||<math>\begin{align}
{{Abgesetzte Formel||<math>\begin{align}
Zeile 6: Zeile 6:
\end{align}</math>}}
\end{align}</math>}}
-
Using de Moivre's formula, the equation can now be written as
+
Mit den Moivreschen Gesetz erhalten wir die Gleichung
{{Abgesetzte Formel||<math>r^5(\cos 5\alpha + i\sin 5\alpha) = \sqrt{2}\Bigl(\cos \frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>r^5(\cos 5\alpha + i\sin 5\alpha) = \sqrt{2}\Bigl(\cos \frac{5\pi}{4} + i\sin\frac{5\pi}{4}\Bigr)\,\textrm{.}</math>}}
-
If we identify the magnitude and argument on both sides, we get
+
Wir vergleichen den Betrag und das Argument der beiden Seiten, und erhalten
{{Abgesetzte Formel||<math>\left\{\begin{align}
{{Abgesetzte Formel||<math>\left\{\begin{align}
Zeile 17: Zeile 17:
\end{align}\right.</math>}}
\end{align}\right.</math>}}
-
(The arguments <math>5\alpha</math> and <math>5\pi/4</math> can differ by a multiple of <math>2\pi</math> and still correspond to the same complex number.)
 
-
This gives that
+
(Die Argumente <math>5\alpha</math> und <math>5\pi/4</math> können sich mit einen Multipel von <math>2\pi</math> unterscheiden, und trotzdem dieselbe komplexe Zahl entsprechen.)
 +
 
 +
Wir erhalten also
{{Abgesetzte Formel||<math>\left\{\begin{align}
{{Abgesetzte Formel||<math>\left\{\begin{align}
Zeile 26: Zeile 27:
\end{align}\right.</math>}}
\end{align}\right.</math>}}
-
If we investigate the argument <math>\alpha</math> more closely, we see that it assumes essentially only five different values,
+
Wir sehen dass das Argument <math>\alpha</math>nur 5 verschiedene Werte annimmt
{{Abgesetzte Formel||<math>\frac{\pi}{4}</math>, <math>\quad\frac{\pi}{4}+\frac{2\pi}{5}</math>, <math>\quad\frac{\pi}{4}+\frac{4\pi}{5}</math>, <math>\quad\frac{\pi}{4}+\frac{6\pi}{5}\quad</math> and <math>\quad\frac{\pi}{4}+\frac{8\pi}{5}</math>}}
{{Abgesetzte Formel||<math>\frac{\pi}{4}</math>, <math>\quad\frac{\pi}{4}+\frac{2\pi}{5}</math>, <math>\quad\frac{\pi}{4}+\frac{4\pi}{5}</math>, <math>\quad\frac{\pi}{4}+\frac{6\pi}{5}\quad</math> and <math>\quad\frac{\pi}{4}+\frac{8\pi}{5}</math>}}
-
since these angle values then repeat to within a multiple of <math>2\pi</math>.
+
Nachdem sich die Winkeln dann wiederholen.
-
In summary, the solutions are
+
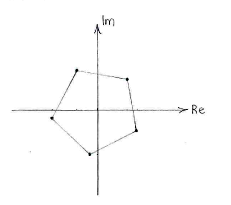
Die Wurzeln sind also
{{Abgesetzte Formel||<math>z = 2^{1/10}\,\Bigl(\cos\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr) + i\sin\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr)\Bigr)\,,</math>}}
{{Abgesetzte Formel||<math>z = 2^{1/10}\,\Bigl(\cos\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr) + i\sin\Bigl(\frac{\pi}{4}+\frac{2n\pi}{5}\Bigr)\Bigr)\,,</math>}}
-
for <math>n=0</math>, <math>1</math>, <math>2</math>, <math>3</math> and
+
für <math>n=0</math>, <math>1</math>, <math>2</math>, <math>3</math> und
<math>4</math>.
<math>4</math>.
[[Image:3_3_2_c.gif|center]]
[[Image:3_3_2_c.gif|center]]

Version vom 15:26, 18. Mai 2009

Wir bringen z und −1−i auf Polarform

z−1−i=r(cos+isin)=2cos45+isin45. 

Mit den Moivreschen Gesetz erhalten wir die Gleichung

r5(cos5+isin5)=2cos45+isin45. 

Wir vergleichen den Betrag und das Argument der beiden Seiten, und erhalten

r55=2=45+2n(n is an arbitrary integer).


(Die Argumente 5 und 54 können sich mit einen Multipel von 2 unterscheiden, und trotzdem dieselbe komplexe Zahl entsprechen.)

Wir erhalten also

r=52=21215=2110=5145+2n=4+52n(n is an arbitrary integer).

Wir sehen dass das Argument nur 5 verschiedene Werte annimmt

4, 4+52, 4+54, 4+56 and 4+58

Nachdem sich die Winkeln dann wiederholen.

Die Wurzeln sind also

z=2110cos4+52n+isin4+52n 

für n=0, 1, 2, 3 und 4.

Von „http://wiki.math.se/wikis/2009/bridgecourse2-TU-Berlin/index.php/L%C3%B6sung_3.3:2c“