Processing Math: Done
3.3:2c alternativ exp
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
| Zeile 1: | Zeile 1: | ||
| - | <math> | + | Wir stellen <math> -1-i</math> exponential dar: |
| - | stelle<math> -1-i</math> exponential dar: | ||
| - | <math>-1-i=r(cos\phi + i sin\phi)=re^{i\phi}</math> wobei r=Betrag und <math>\phi</math>=Argument | + | {{Abgesetzte Formel||<math>-1-i=r(cos\phi + i sin\phi)=re^{i\phi}</math>}} |
| + | wobei r = Betrag und <math>\phi</math> = Argument sind. | ||
| - | <math>r=|-1-i|=\sqrt{(-1)^{2}+(-1)^{2}}=\sqrt{2}</math> | + | {{Abgesetzte Formel||<math>r=|-1-i|=\sqrt{(-1)^{2}+(-1)^{2}}=\sqrt{2}</math>}} |
| - | <math> | + | Dann klammern wir <math> r = \sqrt{2} </math> aus: |
| - | + | {{Abgesetzte Formel||<math>-1-i=\sqrt{2}(-\, \frac{\sqrt{2}}{2} -\, \frac{\sqrt{2}}{2}i)</math>}} | |
| - | <math> | + | Gesucht werden alle Winkel <math>\phi</math>, für die gilt: |
| - | <math>\phi=\frac{\pi}{4}+\pi+2n\pi=\frac{5\pi}{4}+2n\pi</math> mit <math>n \in </math>'''Z''' | ||
| - | + | {{Abgesetzte Formel||<math>cos\phi=\frac{-\sqrt{2}}{2}</math> und <math>sin\phi=\frac{-\sqrt{2}}{2}</math>}} | |
| - | <math>z=\sqrt[\scriptstyle 5]{\sqrt{2}e^{i(\frac{5\pi}{4}+2n\pi)}}=2^{\frac{1}{2}.\frac{1}{5}}e^{i\frac{1}{5}(\frac{5\pi}{4}+2n\pi)}=2^{\frac{1}{10}}e^{i(\frac{\pi}{4}+\frac{2}{5}n\pi)}</math> | + | Diese Winkel sind |
| + | |||
| + | {{Abgesetzte Formel||<math>\phi=\frac{\pi}{4}+\pi+2n\pi=\frac{5\pi}{4}+2n\pi</math>}} | ||
| + | mit <math>n \in </math>'''Z'''. | ||
| + | |||
| + | Also | ||
| + | {{Abgesetzte Formel||<math>z^{5}=-1-i=\sqrt{2}e^{i(\frac{5\pi}{4}+2n\pi)}</math>}} | ||
| + | |||
| + | Wir ziehen die 5. Wurzel, um <math> z </math> aus <math> z^5 </math> zu erhalten: | ||
| + | |||
| + | |||
| + | {{Abgesetzte Formel||<math>z=\sqrt[\scriptstyle 5]{\sqrt{2}e^{i(\frac{5\pi}{4}+2n\pi)}}=2^{\frac{1}{2}.\frac{1}{5}}e^{i\frac{1}{5}(\frac{5\pi}{4}+2n\pi)}=2^{\frac{1}{10}}e^{i(\frac{\pi}{4}+\frac{2}{5}n\pi)}</math>}} | ||
Die Gleichung <math>z^{5}=-1-i</math> hat die Ordnung 5, d.h es gibt 5 verschiedene Lösungen: | Die Gleichung <math>z^{5}=-1-i</math> hat die Ordnung 5, d.h es gibt 5 verschiedene Lösungen: | ||
| Zeile 30: | Zeile 40: | ||
5. <math>n=4</math> : <math>z=2^{\frac{1}{10}}\, e^{i \frac{37\pi}{20} \pi}</math> | 5. <math>n=4</math> : <math>z=2^{\frac{1}{10}}\, e^{i \frac{37\pi}{20} \pi}</math> | ||
| + | |||
| + | Die nächste Lösung für <math> n=5 </math> ist wegen der 2-<math>\pi</math> Periodizität identisch mit der 1. Lösung mit <math> n=0 </math>. | ||
Version vom 13:28, 7. Sep. 2009
Wir stellen
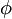 +isin +isin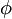 )=rei )=rei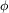 |
wobei r = Betrag und 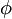
 −1−i −1−i = = (−1)2+(−1)2= (−1)2+(−1)2= 2 2 |
Dann klammern wir  2
2
 2(−2 2(−2 2−2 2−2 2i) 2i) |
Gesucht werden alle Winkel 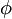
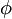 =2− =2− 2 2 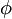 =2− =2− 2 2 |
Diese Winkel sind
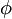 = = 4+ 4+ +2n +2n =45 =45 +2n +2n |
mit 
Also
 2ei(45 2ei(45 +2n +2n ) ) |
Wir ziehen die 5. Wurzel, um
 5 5 2ei(45 2ei(45 +2n +2n )=221 )=221 51ei51(45 51ei51(45 +2n +2n )=2110ei( )=2110ei( 4+52n 4+52n ) ) |
Die Gleichung
1. 

2. 
3. 
4. 
5. 

Die nächste Lösung für 
