Processing Math: Done
Lösung 1.2:2e
Aus Online Mathematik Brückenkurs 2
One way to differentiate the expression could be to expand
To begin with, we have a product of
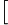 x(2x+1)4 x(2x+1)4 =(x) =(x)  (2x+1)4+x (2x+1)4+x  (2x+1)4 (2x+1)4  =1 =1 (2x+1)4+x (2x+1)4+x  (2x+1)4 (2x+1)4  . . |
We can differentiate the expression
The chain rule then gives
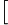 4 4 ddx ddx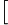 (2x+1)4 (2x+1)4 =4 =4 3 3   =4 =4 (2x+1)3 (2x+1)3 (2x+1) (2x+1) . . |
We carry out the last differentiation directly, and obtain
 =2. =2. |
If we go through the whole calculation from the beginning, it is
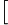 x(2x+1)4 x(2x+1)4 =(x) =(x)  (2x+1)4+x (2x+1)4+x  (2x+1)4 (2x+1)4  =1 =1 (2x+1)4+x (2x+1)4+x 4(2x+1)3 4(2x+1)3 (2x+1) (2x+1) =(2x+1)4+x =(2x+1)4+x 4(2x+1)3 4(2x+1)3 2=(2x+1)4+8x(2x+1)3. 2=(2x+1)4+8x(2x+1)3. |
Both terms contain a common factor
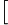 x(2x+1)4 x(2x+1)4 =(2x+1)3 =(2x+1)3 (2x+1)+8x (2x+1)+8x =(2x+1)3(10x+1). =(2x+1)3(10x+1). |
