Lösung 3.3:2a

Aus Online Mathematik Brückenkurs 2

An equation of the type “ zn = a complex number” is called a binomial equation and these are usually solved by going over to polar form and using de Moivre's formula.

We start by writing z and 1 in polar form

z=rcos+isin1=1cos0+isin0 

The equation then becomes

r4cos4+isin4=1cos0+isin0 

where we have used de Moivre's formula on the left-hand side. In order that both sides are equal, they must have the same magnitude and the same argument to within a multiple of 2, i.e.

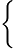r4=14=0+2nn an arbitrary integer  

This means that

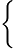r=1=2nn an arbitrary integer  

The solutions are thus (in polar form)

z=1cos2n+isin2n , for n=0 1 2

but observe that the argument on the right-hand side essentially takes only four different values 0 2  and 32, because other values of n give some of these values plus/minus a multiple of 2.

The equation's solutions are therefore

z=1cos0+isin01cos2+isin21cos+isin1cos32+isin32=1i−1−i

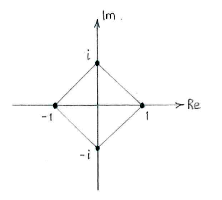
NOTE: note that if we mark these solutions on the complex number plane, we see that they are corners in a regular quadrilateral.