Lösung 3.3:2c

Aus Online Mathematik Brückenkurs 2

We write z and the right-hand side -1-i in polar form

z=rcos+isin-1-i=2cos45+isin45 

Using de Moivre's formula, the equation can now be written as

r5cos5+isin5=2cos45+isin45 

If we identify the magnitude and argument on both sides, we get

r5=25=45+2nn an arbitrary integer  

(The arguments 5 and 45 can differ by a multiple of 2 and still correspond to the same complex number.)

This gives that

r=52=21215=2110=5145+2n=4+52nn an arbitrary integer  

If we investigate the argument more closely, we see that it assumes essentially only five different values,

4 4+52 4+54 4+56 and  4+58

since these angle values then repeat to within a multiple of 2.

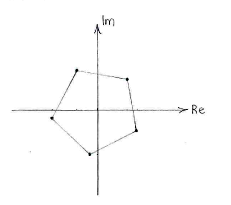
In summary, the roots of the equation are

z=2110cos4+52n+isin4+52n 

for n=0 1 2 3 and 4