Processing Math: Done

Aus Online Mathematik Brückenkurs 2

As usual we begin by completing the square,

z−21+3i2−21+3i2−4+3i=0z−21+3i2−41+23i+49i2−4+3i=0z−21+3i2−41−23i+49−4+3i=0z−21+3i2−2+23i=0

and if we treat as unknown, we have the equation's

w=z−21+3i

Up until now, we have solved binomial equations of this type by going over to polar form, but if we were to do that in this case, we would have a problem with determining the exact value of the argument of the right-hand side. Instead, we put w=x+iy and try to obtain x and y from the equation.

With w=x+iy, the equation becomes

x+iy2=2−23i 

and, with the left-hand side expanded,

x2−y2+2xyi=2−23i

If we set the real and imaginary part of both sides equal, we obtain the equation system

x2−y2=22xy=−23 

We would very well be able to solve this system, but there is a further relation that we can obtain which will simplify the calculations. If we go back to the relation

x+iy2=2−23i 

and take the modulus of both sides, we obtain

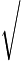x2+y2=22+−232 

i.e.

x2+y2=25

We add this relation to our two other equations:

x2−y2=22xy=−23x2+y2=25

Now, add the first and third equations

EQU1

which gives that y=23. Then, subtracting the first equation from the third,

EQU2

we get y=21. This gives potentially four solutions to the equation system,

x=23y=21x=23y=−21x=−23y=21x=−23y=−21 

but only two of these satisfy the second equation 2xy=−23, namely

x=23y=−21andx=−23y=21 

Hence, we have that the solutions are

w=23−i and w=2−3+i

or, expressed in z,

z=2+i and z=−1+2i

Because the calculation has been rather long, there is the risk that we have calculated incorrectly somewhere and we therefore check that the solutions satisfy the equation in the exercise:

z=2+i:z2−1+3iz−4+3i=2+i2−1+3i2+i−4+3i=4+4i+i2−2+i+6i+3i2−4+3i=4+4i−1−2−7i+3−4+3i=0

z=−1+2i:z2−1+3iz−4+3i=−1+2i2−1+3i−1+2i−4+3i=−12−4i+4i2−−1+2i−3i+6i2−4+3i=1−4i−4+1+i+6−4+3i=0