Processing Math: Done

Aus Online Mathematik Brückenkurs 2

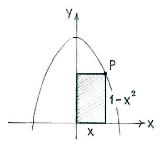
If we call the x-coordinate of the point P x, then its y-coordinate is 1−x2, because P lies on the curve y=1−x2.

The area of the rectangle is then given by

A(x)=(base)(height)=x(1−x2)

and we will try to choose x so that this area function is maximised.

To begin with, we note that, because P should lie in the first quadrant, x0 and also y=1−x20, i.e. x1. We should therefore look for the maximum of A(x) when 0x1.

There are three types of points which can maximise the area function:

1. critical points,
2. points where the function is not differentiable,
3. endpoints of the region of definition.

The function A(x)=x(1−x2) is differentiable everywhere, so item 2 does not apply. In addition, A(0)=A(1)=0, so the endpoints in item 3 cannot be maximum points (but rather the opposite, i.e. minimum points).

We must therefore conclude that the maximum area is a critical point. We differentiate

A(x)=1(1−x2)+x(−2x)=1−3x2

and the condition that the derivative should be zero gives that x=13 ; however, it is only x=13  which satisfies 0x1.

At the critical point, the second derivative A(x)=−6x has the value

A13=−6130

which shows that x=13  is a local maximum.

The answer is that the point P should be chosen so that

P=131−132=1332.