Processing Math: Done
Lösung 3.3:5b
Aus Online Mathematik Brückenkurs 2
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
Complete the square of the left-hand side, | Complete the square of the left-hand side, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\Bigl(z-\frac{2-i}{2}\Bigr)^2-\Bigl(\frac{2-i}{2}\Bigr)^2+3-i &= 0\,,\\[5pt] | \Bigl(z-\frac{2-i}{2}\Bigr)^2-\Bigl(\frac{2-i}{2}\Bigr)^2+3-i &= 0\,,\\[5pt] | ||
\Bigl(z-\frac{2-i}{2}\Bigr)^2-\Bigl(1-i+\frac{1}{4}i^2\Bigr)+3-i&=0\,,\\[5pt] | \Bigl(z-\frac{2-i}{2}\Bigr)^2-\Bigl(1-i+\frac{1}{4}i^2\Bigr)+3-i&=0\,,\\[5pt] | ||
| Zeile 10: | Zeile 10: | ||
Taking the square root then gives that the solutions are | Taking the square root then gives that the solutions are | ||
| - | {{ | + | {{Abgesetzte Formel||<math>z-\frac{2-i}{2} = \pm\frac{3}{2}\,i\quad \Leftrightarrow \quad z=\left\{ \begin{align} |
&1+i\,,\\ | &1+i\,,\\ | ||
&1-2i\,\textrm{.} | &1-2i\,\textrm{.} | ||
Version vom 13:13, 10. Mär. 2009
Complete the square of the left-hand side,
 z−22−i z−22−i 2− 2− 22−i 22−i 2+3−i 2+3−i z−22−i z−22−i 2− 2− 1−i+41i2 1−i+41i2 +3−i +3−i z−22−i z−22−i 2−1+i+41+3−i 2−1+i+41+3−i z−22−i z−22−i 2+49=0 2+49=0 =0 =0 =0 =0 =0. =0. |
Taking the square root then gives that the solutions are
 23i 23i z= z=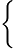 1+i 1+i 1−2i. 1−2i. |
Finally, we substitute the solutions into the equation and check that it is satisfied
 =(1−2i)2−(2−i)(1−2i)+3−i=1−4i+4i2−(2−4i−i+2i2)+3−i=1−4i−4−2+5i+2+3−i=0.
=(1−2i)2−(2−i)(1−2i)+3−i=1−4i+4i2−(2−4i−i+2i2)+3−i=1−4i−4−2+5i+2+3−i=0.
