Processing Math: Done

Aus Online Mathematik Brückenkurs 2

Version vom 09:13, 29. Okt. 2008 (bearbeiten) Tek (Diskussion | Beiträge) K ← Zum vorherigen Versionsunterschied		Aktuelle Version (11:34, 28. Aug. 2009) (bearbeiten) (rückgängig) Sekretariat1 (Diskussion | Beiträge)
(Der Versionsvergleich bezieht 6 dazwischen liegende Versionen mit ein.)
Zeile 1:		Zeile 1:
-	We shall solve the exercise in two different ways.	+	Wir werden das Problem mit zwei verschiedenen Methoden lösen.
-	'''Method 1''' (integration by parts)	+	'''Methode 1''' (partielle Integration)
-	At first sight, integration by parts seems impossible, but the trick is to see the integrand as the product	+	Beim ersten Anblick scheint es unmöglich partielle Integration anzuwenden. Der Trick ist, dass wir den Integrand als das Produkt
-	{{Displayed math||<math>1\centerdot \ln x\,\textrm{.}</math>}}	+	{{Abgesetzte Formel||<math>1\cdot \ln x</math>}}
-	We integrate the factor <math>1</math> and differentiate <math>\ln x</math>,	+	betrachten, <math>1</math> integrieren und <math>\ln x</math> ableiten.
-	{{Displayed math||<math>\begin{align}	+	{{Abgesetzte Formel||<math>\begin{align}
	\int 1\cdot\ln x\,dx		\int 1\cdot\ln x\,dx
	&= x\cdot\ln x - \int x\cdot\frac{1}{x}\,dx\\[5pt]		&= x\cdot\ln x - \int x\cdot\frac{1}{x}\,dx\\[5pt]
	&= x\cdot\ln x - \int 1\,dx\\[5pt]		&= x\cdot\ln x - \int 1\,dx\\[5pt]
-	&= x\cdot\ln x - x + C\,\textrm{.}	+	&= x\cdot\ln x - x + C
	\end{align}</math>}}		\end{align}</math>}}
-	'''Method 2''' (substitution)	+	'''Methode 2''' (Substitution und partielle Integration)
-	It seems difficult to find some suitable expression to substitute, so we try to substitute the whole expression <math>u=\ln x\,</math>. The problem we encounter is how we should handle the change from <math>dx</math> to <math>du</math>. With this substitution, the relation between <math>dx</math> and <math>du</math> becomes	+	Wir substituieren <math>u=\ln x\,</math>. So erhalten wir das Verhältnis
-	{{Displayed math||<math>du = (\ln x)'\,dx = \frac{1}{x}\,dx</math>}}	+	{{Abgesetzte Formel||<math>du = (\ln x)'\,dx = \frac{1}{x}\,dx</math>}}
-	and because <math>u = \ln x</math>, then <math>x=e^u</math> and we have that	+	und da <math>u = \ln x</math>, ist <math>x=e^u</math> und dadurch erhalten wir
-	{{Displayed math||<math>du = \frac{1}{e^u}\,dx\quad\Leftrightarrow\quad dx = e^u\,du\,\textrm{.}</math>}}	+	{{Abgesetzte Formel||<math>du = \frac{1}{e^u}\,dx\quad\Leftrightarrow\quad dx = e^u\,du\,\textrm{.}</math>}}
-	Thus, the substitution becomes	+	Also haben wir
-	{{Displayed math||<math>\begin{align}	+	{{Abgesetzte Formel||<math>\begin{align}
	\int \ln x\,dx		\int \ln x\,dx
	= \left\{\begin{align}		= \left\{\begin{align}
Zeile 39:		Zeile 39:
	\end{align}</math>}}		\end{align}</math>}}
-	Now, we carry out an integration by parts,	+	Dieses Integral berechnen wir durch partielle Integration
-	{{Displayed math||<math>\begin{align}	+	{{Abgesetzte Formel||<math>\begin{align}
	\int u\cdot e^u\,du		\int u\cdot e^u\,du
	&= u\cdot e^u - \int 1\cdot e^u\,du\\[5pt]		&= u\cdot e^u - \int 1\cdot e^u\,du\\[5pt]
	&= ue^u - \int e^u\,du\\[5pt]		&= ue^u - \int e^u\,du\\[5pt]
	&= ue^u - e^u + C\\[5pt]		&= ue^u - e^u + C\\[5pt]
-	&= (u-1)e^u + C\,,	+	&= (u-1)e^u + C
	\end{align}</math>}}		\end{align}</math>}}
-	and the answer becomes	+	und wir erhalten
-	{{Displayed math||<math>\begin{align}	+	{{Abgesetzte Formel||<math>\begin{align}
	\int \ln x\,dx		\int \ln x\,dx
	&= (\ln x-1)e^{\ln x} + C\\[5pt]		&= (\ln x-1)e^{\ln x} + C\\[5pt]
	&= (\ln x-1)x + C\,\textrm{.}		&= (\ln x-1)x + C\,\textrm{.}
	\end{align}</math>}}		\end{align}</math>}}

---

Aktuelle Version

Wir werden das Problem mit zwei verschiedenen Methoden lösen.

Methode 1 (partielle Integration)

Beim ersten Anblick scheint es unmöglich partielle Integration anzuwenden. Der Trick ist, dass wir den Integrand als das Produkt

1lnx

betrachten, 1 integrieren und lnx ableiten.

1lnxdx=xlnx−xx1dx=xlnx−1dx=xlnx−x+C

Methode 2 (Substitution und partielle Integration)

Wir substituieren u=lnx. So erhalten wir das Verhältnis

du=(lnx)dx=x1dx

und da u=lnx, ist x=eu und dadurch erhalten wir

du=1eudxdx=eudu.

Also haben wir

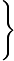lnxdx=udx=lnx=eudu=ueudu.

Dieses Integral berechnen wir durch partielle Integration

ueudu=ueu−1eudu=ueu−eudu=ueu−eu+C=(u−1)eu+C

und wir erhalten

lnxdx=(lnx−1)elnx+C=(lnx−1)x+C.